Log-linearize no-arbitrage condition in a 2-country model

General DSGE Modeling Replication Attempts
1

Hi there,

I would like to log-linearize the no-arbitrage condition between bonds and assets under capital adjustment costs (standard definition like in Boldrin, Christiano, and Fisher (1999)).

scre

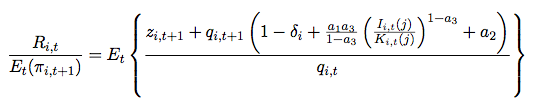
In steady state,

R_ss = Zss + (1-delta)

holds. Furthermore,

qss = 1; inflationSS = 1; a_3 = 1/0.24; a_1 = delta^(a_3); a_2 = delta - (a_1/(1-a_3))(delta)^(1-a_3);

I saw only examples with investment adjustment costs…
Without adjustment costs I have no difficulties to log-linearize the above expression.

In my solution, the adjustment cost term (derivative in the brackets) cancels out when I use Uhlig’s (1999) method. Is this possible?

Thank you very much for any kind of input…

2

Please use the \LaTeX capabilities to provide the solution you derived. That makes it easier to spot problems and does not require to start from scratch.

3

Thank you for your comment. In the attachment you find the pdf version of my question.

Annex_Models.pdf (136.0 KB)

4

Is there no paper available which log-linearizes capital adjustment costs? :thinking: I really need your advise … Many thanks!

5

See https://economics.ca//2007/papers/0207.pdf