Dear Professor Pfeifer,
I’ve got three questions about the model setting when learning the models. Would you please give any advice ? Thank you very much.
(1) I read that the net worth of entrepreneur is generally set as:
{{N}_{t}}=\gamma {{V}_{t}}+W_{t}^{e}
Bernanke et al. set W_{t}^{e} as entrepreneurial wage, and Christiano et al.(2010) set it as initial fund of entrepreneurs. While in the code this W_{t}^{e} is treated as a parameter. Why don’t they directly set it as a parameter in the content?
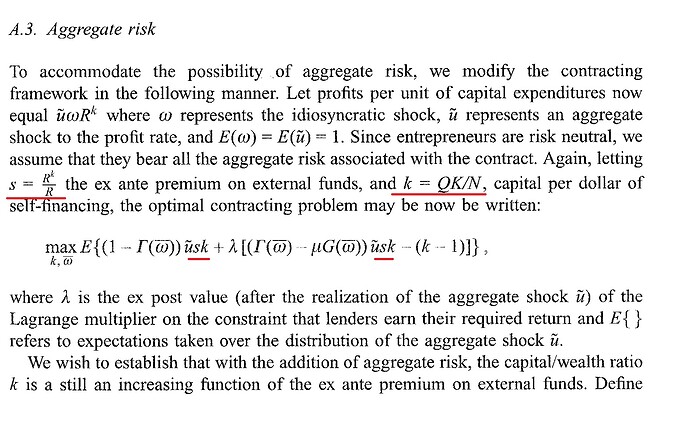
(2) The entrepreneurs’ objective function in BGG(1999) is
\underset{K,\bar{\omega }}{\mathop{\max }}\,\left[ 1-\Gamma \left( {\bar{\omega }} \right) \right]{{R}^{k}}QK
While after setting s={{R}^{k}}/R and k=QK/N, why the objective function become as
\underset{k,\bar{\omega }}{\mathop{\max }}\,\left[ 1-\Gamma \left( {\bar{\omega }} \right) \right]sk ?
(3) In the market clearing part of some papers, the agents are measured with parameters such like {{c}_{t}}=\gamma c_{t}^{P}+\left( 1-\gamma \right)c_{t}^{I}, while in some other papers(e.g. Iacoviello(2010)) the authors just set {{c}_{t}}= c_{t}^{P}+c_{t}^{I}. What is the difference in between?
Thank you for your precious time.