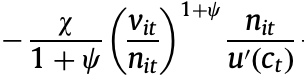Labor search with wage stickiness - convex costs of posting a vacancy alternatives

General DSGE Modeling
1

When introducing wage stickiness in labor search framework, costs of posting a vacancy must no longer be linear but convex, for the following reason. Thomas (2008, p. 940) says:

In the case of staggered wage bargaining, the resulting wage dispersion creates dispersion in the marginal benefit of posting vacancies. If vacancy-posting costs were linear, then the marginal cost of posting vacancies would be the same for all firms and only one firm (the one with the lowest wage) would post vacancies. In order to have an equilibrium where all firms post vacancies, I assume convexity in vacancy-posting costs.

In the same matter Gertler, Sala and Trigari (2008, p. 1720) say:

Because the contract structure leads to temporary wage dispersion and because (to simplify the bargaining problem) we have constant returns at the firm level, quadratic costs are required to keep capital and labor from shifting in mass to the low-wage firms.

How can I see it mathematically? I ask since I don’t fully comprehend how you realize of that, what would happen if I formulate a search labor model with staggered wage bargaining (using Nash solution) and assume that costs of posting a vacancy are linear as usual? Would not there be existence of equilibrium?

Also GalĂ­ (2010, p.20) (although not a fully search labor model, but an arguably very similar one, since he uses the same reason as the previous authors for argumenting the changes) introduce instead of those convex cost in vacancy posting, diminishing returns to scale to labor production:

The assumption of a decreasing returns technology is required in order for wage differentials across firm to be consistent with equilibrium, given the assumption of price taking
behavior (otherwise only the firm with the lowest wage would not be priced out of the
market).

I find Galí’s way more appealing, but I don’t recall where I think having read that introducing diminishing or increasing returns to scale to the production function where the labor that is involved in search frictions enters, causes flaws in Nash bargaining solution since produces different importance of the marginal worker that enters the firm. I mean can Galí’s way be also used in a full labor search model?

If you may help me in understanding this better I’d be very grateful!

2

I haven’t worked with these models, but my hunch is that you would see this during aggregation. But intuitively, the stated results make sense. With wage dispersion, there will be a firm paying the lowest wages. For that firm, hiring additional workers at the lowest wage will be extremely attractive as it will be able to undercut all other firms’ prices, driving them out of the market. That is, unless there is a countervailing force to expanding without limits. There seem to be two ways out: i) convex costs to hiring additional workers or ii) decreasing returns to scale. In the first case, hiring more workers (or posting vacancies for them) will become increasingly more expensive. In the second case, additional workers will become less productive for the firm.

1 Like
3

Thanks!! That makes a lot of sense. Also may you have any idea why Thomas divides the vacancy costs by the marginal utility?
image

I think it may have to do with the SDF, but I don’t see why this u’(c) would enter here.

4

I guess it has to do with the assumption on page 940 at the top

This comes at a utility cost for the management

1 Like
5

Indeed, but you see any reason for including it? I mean if I were to drop it would it make any substantial difference? Thanks!!

6

That’s hard to tell. The nice thing about utility costs is that they do not influence the resource constraint. They are purely internal.

1 Like
7

Indeed that’s very useful, and actually I didn’t know it, but how does that happen (that vacancy posting costs do not appear in resource constraint)? Since in the algebra those costs will still appear, right? or is it the case where if I assume utility costs, I have the ability to make disappear those costs from resource constraint? Thanks

8

This is by assumption. If costs are purely in terms of utility instead of goods, then they will of course not show up in the resource constraint. The disutility from working does not show up there either.

1 Like
9

That’s really useful. Thanks!!