A question about steady state

Steady state
1

Hi everyone.
I have some questions about a paper.

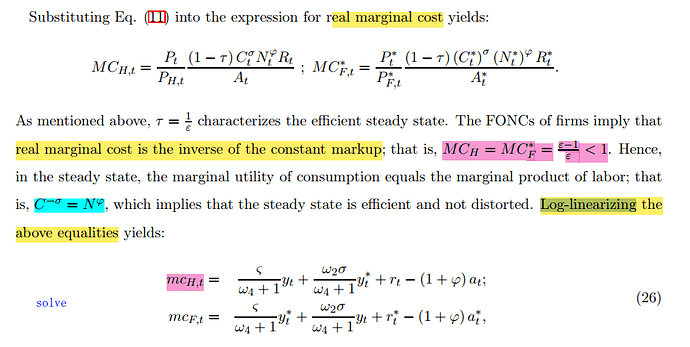
  1. Could anyone tell me why the equation C^{-\sigma}=N^\varphi [colored in blue in the following picture] is established? What happened to P, R, and A?
    I think in the steady state, R and A will stay at 1 and P_t/P_{H,t}=1, but I am not sure.
  2. Will the Log-linearizing version of MC equal to zero and the Log-linearizing version of A become zero, too? Because MC and A become a constant illustrated above. If I am wrong, what will happen to equation (26) insteady state?
    1665455030662
    1665455030662924×465 64.2 KB

    Thanks for any suggeations! Best wishes to you!

Here is the paper:
3.Tae-Seok, Eiji, 2013, Productivity shocks and monetary policy in a two-country model, Dynare working papers.pdf (348.3 KB)

2
  1. I haven’t checked all computations. But the price ratio P_t/P_{H,t} is typically normalized to 1 as is TFP A. But I don’t know what happened to R_t.
  2. In steady state all variables as deviations from steady state are 0. But that does not help you in computing the steady state of the level variables.
3

Thank you for your reply, professor jpfeifer!
Here are my thought and question:

1.The Log-linearizing version of R is an endogenous variable, so I think the same thing happened to R as A and P,so R will stay at 1. I am not sure, but I think so.
According to the papper: 1/R(t) = Q(t,t+1) = C(t+1)^(-sigma)*P(t) / (P(t+1)*C(t)^(-sigma))

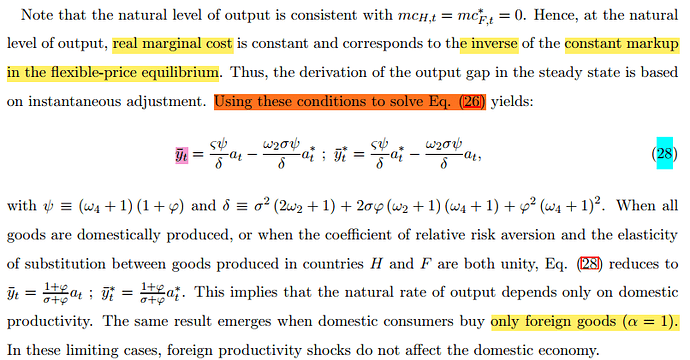
2.During the computations from (26) to (28), I also feel confuse about what happened to r(t), it is disappeared. I can get the numerator of (28) without the help of r(t), so I guess is combined with y(t) and delte, but I have no idea of how to do that. Could you please offer me some suggeations to eliminate r(t) and get delte?

1665561916186
1665561916186806×421 69.1 KB

Once again, thanks for your help many times!

4
  1. R in steady state should be 1/\beta. That’s what I find strange.
  2. Why are you linearizing the model. It seems easier to work with the nonlinear one.
5

To be honest, I am still confused about which fomulars in the model should be posted in dynare, so I can only follow the paper’s idea to learn something about the relationship between dynare code and model.
And I find a easier fomular which can be used to repalce the fomular(28) in dynare to solve this problem.
Thanks for your help! Best wishes to you!