I’m having trouble aggregating production of intermediate firms (indexed by j\in[0,1]) into aggregate production. Concretely, in the case with no capital intermediate production of the j-firm is: Y_t(j)=A_t N_t(j). Given demand for j-firm intermediate good Y_t(j)=\left(\frac{P_t(j)}{P_t}\right)^{-\epsilon}Y_t, where no index means production or price level of the final firm as usual. Integrating in both sides for aggregating we get:
\int_{0}^{1}Y_t(j)dj=Y_t\int_{0}^{1}\left(\frac{P_t(j)}{P_t}\right)^{-\epsilon}dj
Substituting j-firm production function:
A_t\int_{0}^{1}N_t(j)dj=Y_t\int_{0}^{1}\left(\frac{P_t(j)}{P_t}\right)^{-\epsilon}dj
Market-clearing implies N_t=\int_0^1N_t(j)dj, then we can reduce to: A_tN_t=Y_t\int_{0}^{1}\left(\frac{P_t(j)}{P_t}\right)^{-\epsilon}dj.
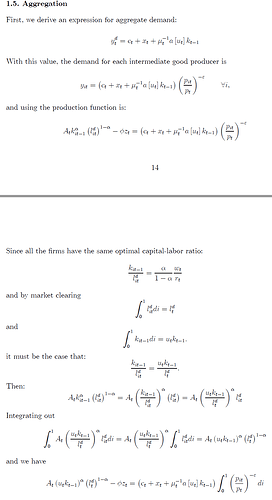
In a case with capital (assuming Cobb-Douglas technology for intermediate firms): \int_{0}^{1}Y_t(j)dj=Y_t\int_{0}^{1}\left(\frac{P_t(j)}{P_t}\right)^{-\epsilon}dj\implies A_t\int_{0}^{1}K_t(j)^{\alpha}N_t(j)^{1-\alpha}dj=Y_t\int_{0}^{1}\left(\frac{P_t(j)}{P_t}\right)^{-\epsilon}dj. Where similarly to the case with no capital, K_t(j) and N_t(j) are j-firm demand for each input. How should the LHS of the equation be aggregated?
My try: \int_{0}^{1}K_t(j)^{\alpha}N_t(j)^{1-\alpha}dj=K_t^{\alpha}N_t^{1-\alpha}. Where K_t and N_t are aggregate supply of inputs. I think this is correct, but I’m not able to “prove” it without just assuming it, since it’s not as clear as in the no-capital case, given the Cobb-Douglas form.